Equation of a Circle In geometry, a circle can also be represented in the expanded formIt is the result of expanding the binomial squares in the standard form and combining like terms To find the equation of circle we use the distance formulaThe last equation graphs a parabola How To Graph a Circle Equation A circle can be thought of as a graphed line that curves in both its x and y values This may sound obvious, but consider this equation y = x 2 4The second equation graphs a straight line;

How To Find The Equation Of A Circle Sat Math
Circle equation x^2+y^2=r^2
Circle equation x^2+y^2=r^2-Suppose mathf(x,y) = x^2 y^2/math Let's look at the partial derivatives of this function math\displaystyle\frac{\partial f}{\partial x}= 2x/math mathPythagoras Pythagoras' Theorem says that for a right angled triangle, the square of the long side equals the sum of the squares of the other two sides x 2 y 2 = 1 2 But 1 2 is just 1, so x 2 y 2 = 1 (the equation of the unit circle) Also, since x=cos and y=sin, we get (cos(θ)) 2 (sin(θ)) 2 = 1 a useful "identity" Important Angles 30°, 45° and 60° You should try to remember
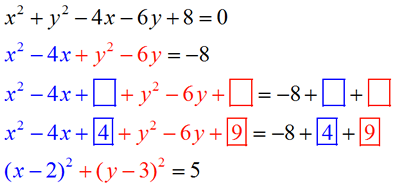


Circle Equations Mathbitsnotebook Geo Ccss Math
Find the center and radius, then graph x2 y2 −4x−6y4 =0 x 2 y 2 − 4 x − 6 y 4 = 0 We need to rewrite this general form into standard form in order to find the center and radius x2 y2 −4x−6y4 = 0 x 2 y 2 − 4 x − 6 y 4 = 0 Group the xterms and yterms Collect the constants on the right right sideIn the diagram, a circle centered at the origin, a right triangle, and the Pythagorean theorem are used to derive the equation of a circle, x^2 y^2 = r^2 If the center of the circle were moved from the origin to the point (h, k) and point P at (x, y) remains on the edge of the circle, which could represent the equation of the new circle? · Example 1 Find the area enclosed by the circle 𝑥2 𝑦2 = 𝑎2 Drawing circle 𝑥^2 𝑦^2= 𝑎^2 Center = (0, 0) Radius = 𝑎 Hence OA = OB = Radius = 𝑎 A = (𝑎, 0) B = (0, 𝑎) Since Circle is symmetric about xaxis and yaxis Area of circle = 4 × Area of Region OBAO = 4 ×
Tangent to a Circle with Center the Origin A standard circle with center the origin (0,0), has equation x 2 y 2 = r 2 Where r is the circle radius Examples (11) A circle has equation x 2 y 2 = 34 The point A (5,3) lies on the edge of the circleWhere there is a Tangent line touching, along with a corresponding Normal lineExample 1 Find the points of intersection of the circles given by their equations as follows (x 2) 2 (y 3) 2 = 9 (x 1) 2 (y 1) 2 = 16 Solution to Example 1 We first expand the two equations as follows x 2 4x 4 y 2 6y 9 = 9 x 2 2x 1 y 2 2y 1 = 16 Multiply all terms in the first equation by 1 to obtain an equivalent equation and keep the second equationX 2 y 2 = r 2 where x,y are the coordinates of each point and r is the radius of the circle In its simplest form, the equation of a circle is What this means is that for any point on the circle, the above equation will be true, and for all other points it will not
Write the equation for the circle with center at ( 8, 6) and radius of 10 (x8)² (y 6)² = 10 (x8)² (y 6)² = 100 (x8)² (y 6)² = 100 Find the standard equation for the circle with center on the positive xaxis and passing through the origin with radius of 2 x² y² = 4 (x 2)² y² = 4 (x 2)² y² = 4 Choose the appropriate description for the equation Given$ x^2 y^2 $ $ x $ $ y $ $ = 0 $ NOTE To input square root symbol type letter 'r' For example $\color{blue}{\text{ r13 } = \sqrt{13}} $ ,$\color{blue}{\text{ 2r3 } = 2\sqrt{3}} $ ,$\color{blue}{ \text{ 2r(3/5) }= 2 \sqrt{\frac{3}{5}}} $This video explains how to write the general form of a circle in standard form and then graph the circlehttp//mathispower4ucom


How To Reduce The Equation To Standard Form And Find The Center And Radius Of The Circle X 2 Y 2 14x 6y 33 Quora



How To Find The Equation Of A Circle Sat Math
Stack Exchange network consists of 176 Q&A communities including Stack Overflow, the largest, most trusted online community for developers to learn, share their knowledge, and build their careers Visit Stack Exchange0726 · This means that, using Pythagoras' theorem, the equation of a circle with radius r and centre (0, 0) is given by the formula \(x^2 y^2 = r^2\) Example Find the equation of a circleThis of the form x 2 y 2 Ax By C = 0 where A = 4, B = 6, C = 9 Hence, the general form of the circle equation is x 2 y 2 – 4x – 6y 9 = 0 FORMULAS Related Links
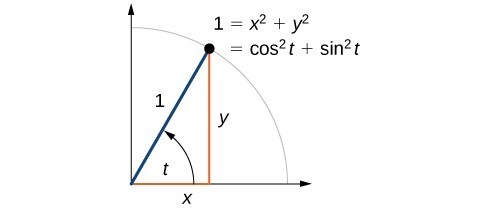


Unit Circle Sine And Cosine Functions Precalculus Ii



Circle Equations
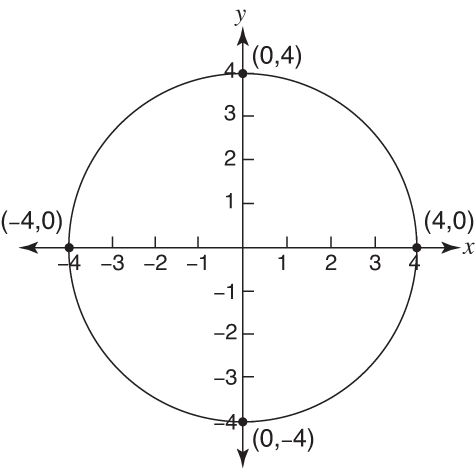
· To obtain the plot points manipulate the equation as below Given x2 y2 = r2 → x2 y2 = 4 Subtract x2 from both sides giving y2 = 4 −x2 Take the square root of both sides y = √4 − x2 Now write it as y = ± √4 −x2 '~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~Free Circle calculator Calculate circle area, center, radius and circumference stepbystep This website uses cookies to ensure you get the best experience circleequationcalculator radius x^26x8yy^2=0 en Related Symbolab blog posts My Notebook, the Symbolab waySuppose we have a circle with the equation x^2 y^2 =25 What is the equation to the tangent to the circle at point (4,3)?


Midpoint Formula Distance Formula X 1 Y 1 X 2 Y 2 1 3 2 And 7 8 2 2 5 And 4 10 1 1 2 And 4 6 2 2 5 And 3 7 Coordinate Ppt Download


Solution Sketch The Circle Whose Equation Is X2 Y2 100 Using The Same System Of Coordinate Axes Graph The Line X 3y 10 Which Should Intersect The Circle Twice At A 10 0
Examples x^2y^2=1 radius\x^26x8yy^2=0 center\(x2)^2(y3)^2=16 area\x^2(y3)^2=16 circumference\(x4)^2(y2)^2=25 circleequationcalculator x^2y^2X 2 y 2 = r 2 Subtract y^ {2} from both sides Subtract y 2 from both sides x^ {2}=r^ {2}y^ {2} x 2 = r 2 − y 2 Take the square root of both sides of the equation Take the square root of both sides of the equation x=\sqrt {\left (ry\right)\left (yr\right)} x=\sqrt {\left (ry\right)\left (yr\right)}A line is tangent to the circle x^2y^2=41, at the point (4,5) find the slope of the radius from the center to the point of tangencywrite the equation of the line tangent



Circle


Circle
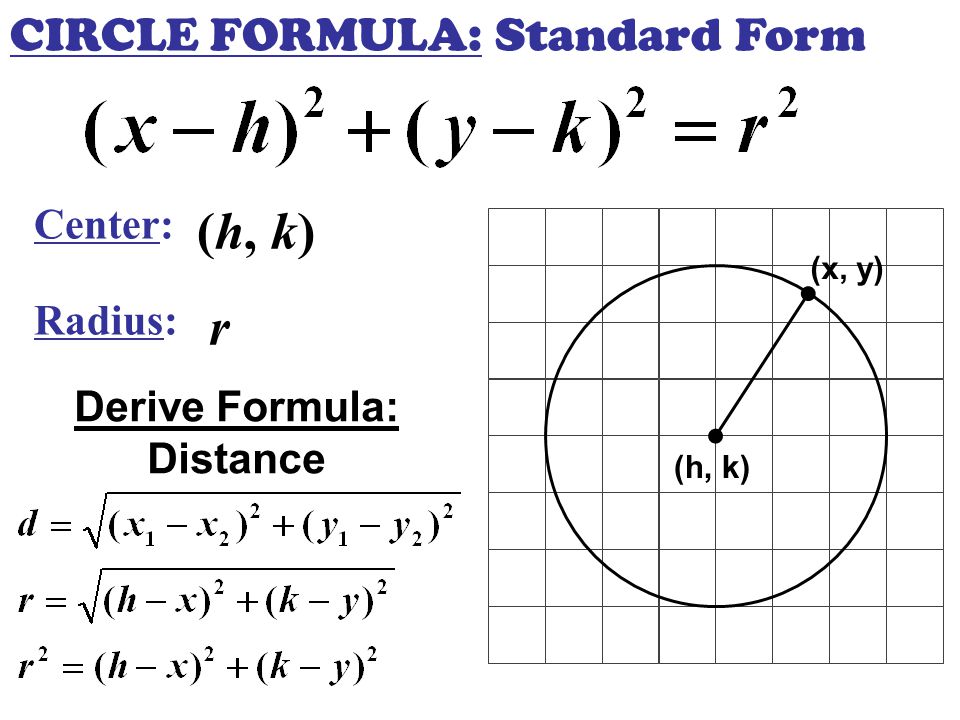
The equation of the circle concentric with the circle x 2 y 2 2gx 2fy c = 0 is of the form x 2 y 2 2gx 2fy k = 0 The equation of the concentric circles differs by constant onlyThe standard equation of a circe is in the form (x h) 2 (y k) 2 = r 2Where (h, k) is the cordinate of the center and r is the radius The given equation, x 2 y 2 12y = 0 can also be written as x 2 0x y 2 12y = 0 Subtract from both sidesThis allows us to use the Pythagorean Theorem to find that the equation for this circle in standard form is x 2 y 2 = r 2 This is true for any point on the circle since any point on the circle is an equal distance, r, from the center Centered at any location To find the equation for a circle in the coordinate plane that is not centered at the origin, we use the distance formula



Equation Of A Sphere Expii


Solved Identify The Center And Radius Of The Circle With The Equation X 5 2 Y 12 2 25 Center Radius 2 Identify Course Hero


